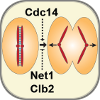
|
Comprehensive Model of the Budding Yeast Cell Cycle
We modify the regulation of mitotic exit to include the roles of Cdc5 and MEN pathway
in triggering Cdc14 release. We integrate both the START and the mitotic exit modules into a full budding
yeast model. Our model provides a unified account of the observed phenotypes of 257 mutant strains (98% of
the data set used to constrain the model) and new insights into how the cell division cycle is regulated in
budding yeast.
|
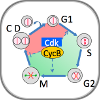
|
Systems Biology of Cell Cycle Control in Eukaryotes - The cell division cycle (G1->S->G2->M->G1) is not an autonomous oscillator; the transitions between stages are guarded by checkpoints and behave like irreversible bistable switches. We study how such switches emerge from the network and how checkpoint mechanisms use them to maintain genomic integrity.
|
|
Functional Motifs in Cellular Regulatory Networks - Complex control networks can be decomposed into simple motifs that carry out
specific functions in a cell, such as signal transduction, noise suppression, homeostasis, oscillations, toggle switch, logic gate, cock-and-fire, and adaptation. We use
bifurcation theory
to analyze these motifs.
|

|
The "Standard Component Modeling" Strategy - We assign components according to their time scales into three classes of variables, each described by a different rate law: ODE for protein synthesis/degradation, soft-Heaviside function H(x)=1/(1+exp(-x)) for post-translational modification, and algebraic equation for complex formation. They then serve as building blocks for modeling complex protein networks.
|

|
Budding Yeast
Model - The molecular machinery of eukaryotic cell cycle control is known in
greater detail for the budding yeast, Saccharomyces cerevisiae, than for any
other organism. This mathematical model helps build confidence in our current
understanding while giving direction for future study.
|
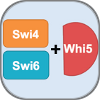
|
Model of the START Transition in the budding yeast - We have updated the 2004 budding yeast model (Mol. Biol. Cell 15:3841) to include a more detailed and accurate descripiton of the START transition. We modeled the regulation of SBF and MBF by Cdk kinases, Bck2, and Whi5, and the effect of phosphorylation of Whi5, Swi4, and Swi6 on the timing of the START transition.
|
|
Generic Cell Cycle Model
- This is a mathematical model of the conserved control mechanisms of eukaryotic
cells, including budding yeast, fission yeast, Xenopus embyos and mammalian cells.
|

|
Automatic Parameter Estimation for the Budding Yeast Model - This is a challenging problem - the control network is complex with 100+ variables and 200+ parameters, and the data available (viabilities of mutants) are qualitative. We devise a strategy combining Latin Hypercube Sampling and Genetic Algorithm. Our approach yields fruitful results.
|

|
Stochastic Models of the Budding Yeast Cell Cycle -
Moleuclar noises can have a significant effect on the dynamic properties of a regulatory network. We are evaluating approximation methods for the standard Gillespie's algotithm for efficient stochastic simulation, using the budding yeast cell cycle control network as a test case.
|

|
Estrogen Signaling in Breast Cancer Cells - We present preliminary mathematical models of the basic decision circuits of cell cycle regulation in breast cancer cells. Such models
(Tavassoly et al. 2015,
Chen et al. 2014,
2013,
Parmar et al. 2013,
Clarke et al. 2012,
Tyson et al. 2011)
may aid our understanding of their susceptibility or resistance to endocrine therapy.
|

|
T Cell Differentiation - Pathogen-driven differentiation of CD4+ T cells is often heterogeneous in terms of the induced phenotypic diversity. At least four distinct lineages play diverse roles in the immune system. Our model can reproduce known properties of differentiation of CD4+
T cells, such as heterogeneous differentiation of TH1-TH2, TH1-TH17 and iTReg-TH17 under single or mixed types of stimuli.
|

|
The Core Model of Caulobacter crescentus Cell Cycle Control is conjectured as a CtrA master-regulator switch that drives the asymmetric cell division. We further investigate the role of dynamical localization of DivL and PleC in the establishment of cellular asymmetry.
|

|
Circadian Rhythm Database
- We surf around the molecular basis of the circadian rhythm of the fruit fly, and
see how the experimental data can be put together as a model, and simulated.
|

|
PET - The parameter estimation toolkit pushes the envelope of systems biology software tools by providing cutting edge technology for mathematical simulations and parameter estimation.
|

|
JigCell - Problem-solving environments can greatly facilitate the development of complex models of biological systems. JigCell is both a platform for
experimenting with software solutions that aid computational biologists as well
as a practical environment that currently benefits the computational biologist.
|
















